In the last section, we dug further into how equations in different forms are related to graphs. In this section, we are going to investigate this even more by comparing the effects of different slopes. We will do this by determining when two equations result in lines might be parallel or perpendicular to each other, or neither!
First, let's recall what the slope tells us intuitively: it describes the "steepness" of a line. This means that we can look at a slope for an equation and have some expectations for what the corresponding graph might look like:
Because slope controls the “steepness” of a line, it is a simple matter to see that parallel lines must have the same slope.
Let \(\boldsymbol_\) be a line having slope \(m_\). Let \(\boldsymbol_\) be a line having slope \(m_\). If \(\boldsymbol_\) and \(\boldsymbol_\) are parallel, then
That is, any two parallel lines have the same slope.
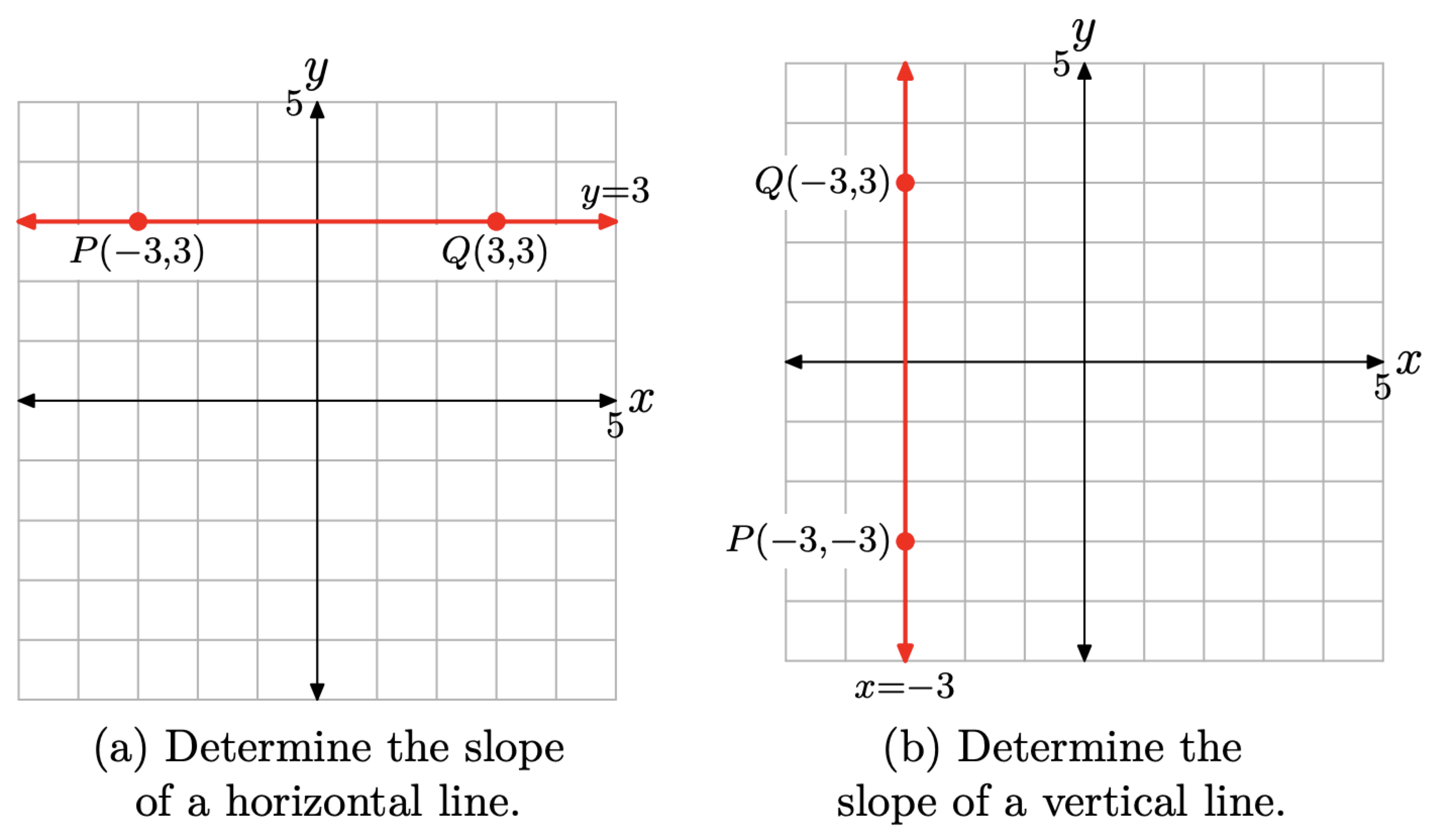
What is the slope of any horizontal line? What is the slope of any vertical line?
Solution
One would expect that our definition would verify that the slope of any horizontal line is zero. Select, for example, the horizontal line shown in Figure 9(a). Select the points (−3, 3) and (3, 3) on this line.

With \(\left(x_, y_\right)=(-3,3)\) and \(\left(x_, y_\right)=(3,3)\)
Thus, the horizontal line in Figure \(\PageIndex\)(a) has slope equal to zero, exactly as expected. Further, all horizontal lines are parallel to this horizontal line and have the same slope. Therefore, all horizontal lines have slope zero.
We would surmise that the vertical line in Figure \(\PageIndex\)(b) has undefined slope (we’ll explore this more fully in the exercises). In Figure \(\PageIndex\)(b), we’ve selected the points P(−3, −3) and Q(−3, 3) on the vertical line. With \(\left(x_, y_\right)=P(-3,-3)\) and \(\left(x_, y_\right)=Q(-3,-3)\), \[\text < Slope >=\frac=\frac
The slope of the vertical line in Figure \(\PageIndex\)(b) is undefined because division by zero is meaningless. Further, all vertical lines are parallel to this vertical line and have undefined slope.
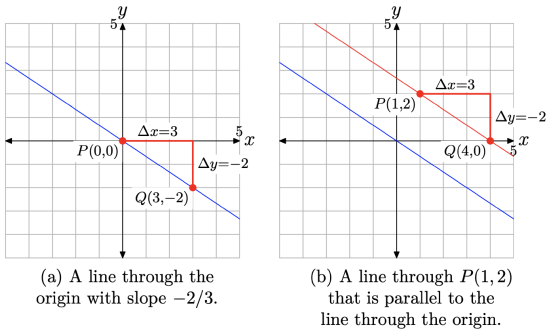
Draw a line through the point P(1, 2) that is parallel to the line passing through the origin with slope −2/3.
Solution
We will first draw a line through the origin with slope −2/3. Plot the point P(0, 0), then move 3 units to the right and 2 units downward to the point Q(3, −2), as shown in Figure \(\PageIndex\)(a). Draw a line through the points P and Q as shown in Figure \(\PageIndex\)(a).
Next, plot the point P(1, 2) as shown in Figure \(\PageIndex\)(b). To draw a line through this point that is parallel to the line through the origin, this second line must have the same slope as the first line. Therefore, start at the point P(1, 2), as shown in Figure \(\PageIndex\)(b), then move 3 units to the right and 2 units downward to the point Q(4, 0). Draw a line through the points P and Q as shown in Figure \(\PageIndex\)(b). Note that this second line is parallel to the first.
The relationship between the slopes of two perpendicular lines is not as straightforward as the relation between the slopes of two parallel lines. Let’s begin by stating the pertinent property.
Let \(L_\) be a line having slope \(m_\). Let \(L_\) be a line having slope \(m_\). If \(L_\) and \(L_\) are perpendicular, then
That is, the product of the slopes of two perpendicular lines is −1.
We can solve equation (14) for \(m_\) in terms of \(m_\).
Equation (15) tells us that the slope of the first line is the negative reciprocal of the slope of the second line.
For example, suppose that \(L_\) and \(L_\) are perpendicular lines with slopes \(m_\) and \(m_\), respectively
Note that in each bulleted item, the product of the slopes is −1.
We won’t provide a proof of equation (15), but we will provide some motivating evidence in the form of a graph.
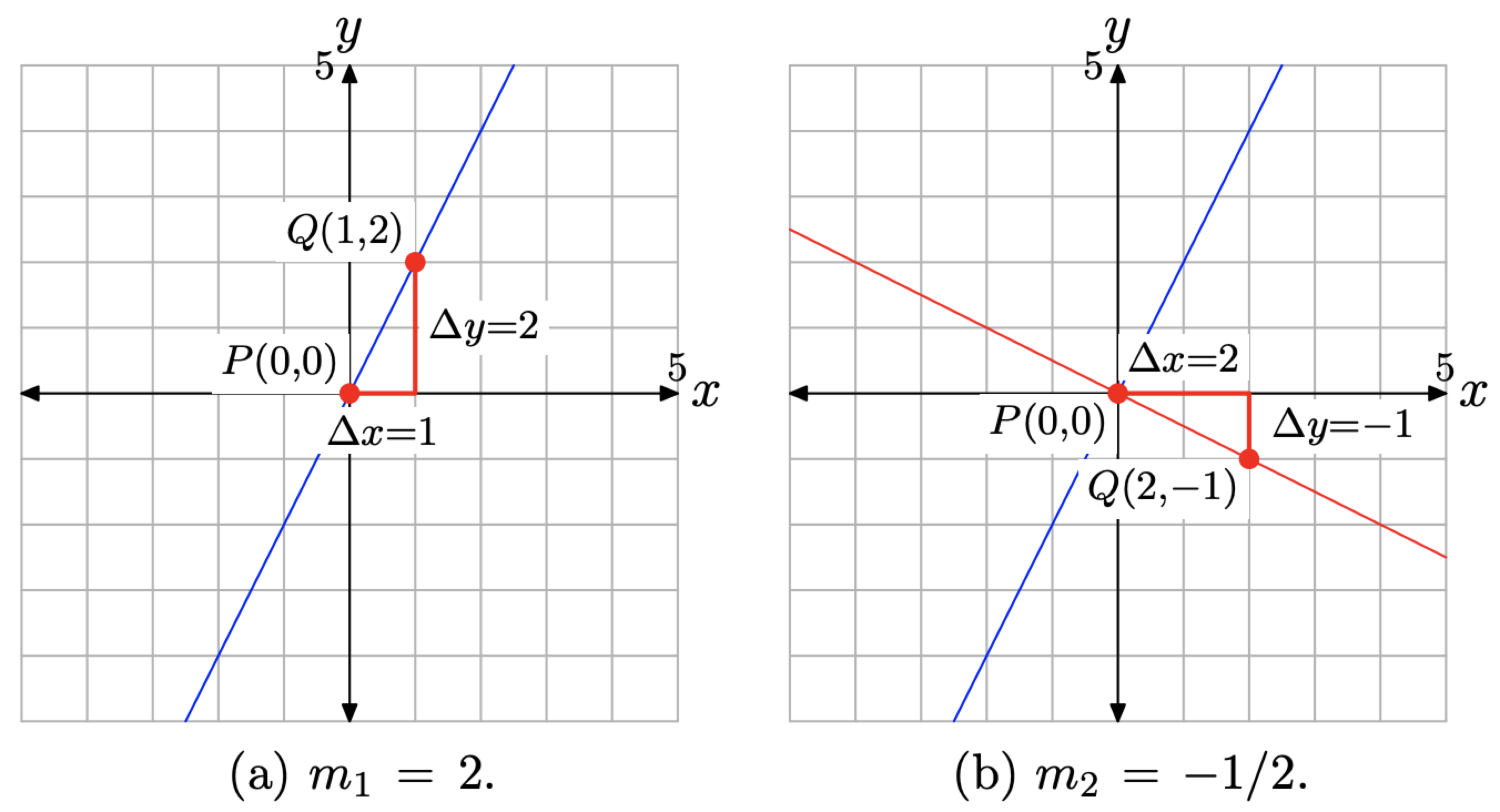
Sketch the graphs of the lines passing through the origin having slopes 2 and −1/2.
Solution
In Figure \(\PageIndex\)(a), we’ve plotted the point P(0, 0) at the origin, then moved 1 unit to the right and 2 units upward to the point Q(1, 2). The resulting line passes through the origin and has slope \(m_=2\) (alternatively, \(m_=2 / 1\)).
In Figure \(\PageIndex\)(b), we’ve again plotted the point P(0, 0) at the origin, then moved 2 units to the right and 1 unit downward to the point Q(2, −1). The resulting line passes through the origin and has slope \(m_=-1 / 2\).
There are two important points that need to be made about the lines in Figure \(\PageIndex\)(b).
The two lines in Figure \(\PageIndex\)(b) are perpendicular. They meet and form a right angle of \(90^\). If you have a protractor available, you might want to measure the angle between the two lines and note that the measure of the angle is \(90^\).
The product of the two slopes is
Since there are very specific rules about the slopes of lines that are parallel or perpendicular to each other, this makes it possible to use the equation of a line to determine the equation of another line that is parallel or perpendicular to it. Let's start with when we want to find a line parallel to another line.
Suppose we need to find an equation of a line that passes through a specific point and is parallel to a given line. We can use the fact that parallel lines have the same slope. So we will have a point and the slope—just what we need to use the point-slope equation.
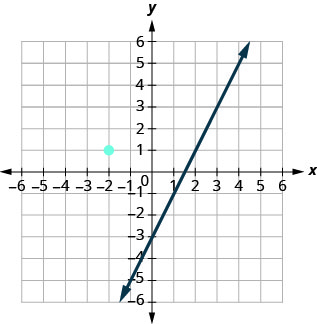
First, let’s look at this graphically.
This graph shows \(y=2x−3.\) We want to graph a line parallel to this line and passing through the point \((−2,1)\).
 negative 8 to 8. The line goes through the points (0, negative 3), (1, negative 1), and (2, 1). The point (negative 2, 1) is plotted. The line does not go through the point (negative 2, 1)." />
negative 8 to 8. The line goes through the points (0, negative 3), (1, negative 1), and (2, 1). The point (negative 2, 1) is plotted. The line does not go through the point (negative 2, 1)." />
We know that parallel lines have the same slope. So the second line will have the same slope as \(y=2x−3\). That slope is \(m_∥=2\). We’ll use the notation m∥m∥ to represent the slope of a line parallel to a line with slope m. (Notice that the subscript || looks like two parallel lines.)
The second line will pass through \((−2,1)\) and have \(m=2\).
To graph the line, we start at\((−2,1)\) and count out the rise and run.
With \(m=2\) (or \(m=\frac\)), we count out the rise 2 and the run 1. We draw the line, as shown in the graph.
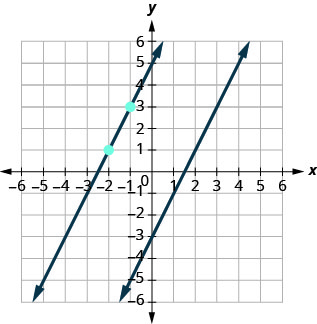 negative 8 to 8. The first line goes through the points (0, negative 3), (1, negative 1), and (2, 1). The points (negative 2, 1) and (negative 1, 3) are plotted. The second line goes through the points (negative 2, 1) and (negative 1, 3)." />
negative 8 to 8. The first line goes through the points (0, negative 3), (1, negative 1), and (2, 1). The points (negative 2, 1) and (negative 1, 3) are plotted. The second line goes through the points (negative 2, 1) and (negative 1, 3)." />
Do the lines appear parallel? Does the second line pass through\((−2,1)\)?
We were asked to graph the line, now let’s see how to do this algebraically.
We can use either the slope-intercept form or the point-slope form to find an equation of a line. Here we know one point and can find the slope. So we will use the point-slope form.
How to Find the Equation of a Line Parallel to a Given Line and a Point
Find an equation of a line parallel to \(y=2x−3\) that contains the point \((−2,1)\). Write the equation in slope-intercept form.
Answer
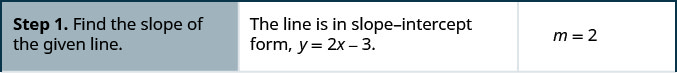 the slope of the given line. The line is in slope-intercept form, y equals 2 x minus 3. m equals 2." />
the slope of the given line. The line is in slope-intercept form, y equals 2 x minus 3. m equals 2." /> the slope of the parallel line. Parallel lines have the same slope. m equals 2." />
the slope of the parallel line. Parallel lines have the same slope. m equals 2." />


Look at graph with the parallel lines shown previously. Does this equation make sense? What is the y-intercept of the line? What is the slope?
Find an equation of a line parallel to the line \(y=3x+1\) that contains the point \((4,2)\). Write the equation in slope-intercept form.
Answer
Find an equation of a line parallel to the line \(y=12x−3\) that contains the point \((6,4)\).
Write the equation in slope-intercept form.
Answer
Now, let’s consider perpendicular lines. Suppose we need to find a line passing through a specific point and which is perpendicular to a given line. We can use the fact that perpendicular lines have slopes that are negative reciprocals. We will again use the point-slope equation, like we did with parallel lines.
This graph shows \(y=2x−3\). Now, we want to graph a line perpendicular to this line and passing through \((−2,1)\).
 negative 8 to 8. The line goes through the points (0, negative 3), (1, negative 1), and (2, 1). The point (negative 2, 1) is plotted. The line does not go through the point (negative 2, 1)." />
negative 8 to 8. The line goes through the points (0, negative 3), (1, negative 1), and (2, 1). The point (negative 2, 1) is plotted. The line does not go through the point (negative 2, 1)." />
We know that perpendicular lines have slopes that are negative reciprocals.
We’ll use the notation \(m_⊥\) to represent the slope of a line perpendicular to a line with slope m. (Notice that the subscript \(⊥\) looks like the right angles made by two perpendicular lines.)
\[y=2x−3 perpendicular line \nonumber\]
We now know the perpendicular line will pass through \((−2,1)\) with \(m⊥=−12\).
To graph the line, we will start at \((−2,1)\) and count out the rise \(−1\) and the run \(2\). Then we draw the line.
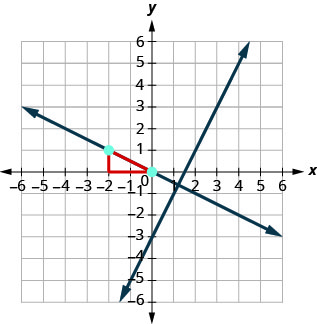 negative 8 to 8. The first line goes through the points (0, negative 3), (1, negative 1), and (2, 1). The points (negative 2, 1) and (0, 0) are plotted. A right triangle is drawn connecting the points (negative 2, 1), (negative 2, 0), and (0, 0). The second line goes through the points (negative 2, 1) and (0, 0)." />
negative 8 to 8. The first line goes through the points (0, negative 3), (1, negative 1), and (2, 1). The points (negative 2, 1) and (0, 0) are plotted. A right triangle is drawn connecting the points (negative 2, 1), (negative 2, 0), and (0, 0). The second line goes through the points (negative 2, 1) and (0, 0)." />
Do the lines appear perpendicular? Does the second line pass through\((−2,1)\)?
We were asked to graph the line, now, let’s see how to do this algebraically.
We can use either the slope-intercept form or the point-slope form to find an equation of a line. In this example we know one point, and can find the slope, so we will use the point-slope form.
How to Find the Equation of a Line Perpendicular to a Given Line and a Point
Find an equation of a line perpendicular to \(y=2x−3\) that contains the point \((−2,1)\). Write the equation in slope-intercept form.
Answer  the slope of the given line. The line is in slope-intercept form, y equals 2 x minus 3. m equals 2." />
the slope of the given line. The line is in slope-intercept form, y equals 2 x minus 3. m equals 2." />  the slope of the perpendicular line. The slopes of perpendicular lines are negative reciprocals. m equals negative 1 divided by 2" />
the slope of the perpendicular line. The slopes of perpendicular lines are negative reciprocals. m equals negative 1 divided by 2" /> 
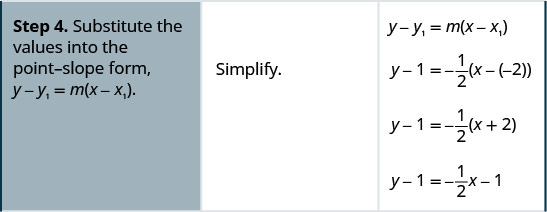

Find an equation of a line perpendicular to the line \(y=3x+1\) that contains the point \((4,2)\). Write the equation in slope-intercept form.
Answer
Find an equation of a line perpendicular to the line \(y=12x−3\) that contains the point \((6,4)\). Write the equation in slope-intercept form.
Answer
Find an equation of a line perpendicular to \(x=5\) that contains the point \((3,−2)\). Write the equation in slope-intercept form.
Answer
Again, since we know one point, the point-slope option seems more promising than the slope-intercept option. We need the slope to use this form, and we know the new line will be perpendicular to x=5.x=5. This line is vertical, so its perpendicular will be horizontal. This tells us the m⊥=0.m⊥=0.
Identify the point.Identify the slope of the perpendicular line.Substitute the values intoy−y1=m(x−x1).Simplify.(3,−2)m⊥y−y1y−(−2)y+2y=====0m(x−x1)0(x−3)0−2Identify the point.(3,−2)Identify the slope of the perpendicular line.Substitute the values intoy−y1=m(x−x1).Simplify.m⊥=0y−y1=m(x−x1)y−(−2)=0(x−3)y+2=0y=−2
Sketch the graph of both lines. On your graph, do the lines appear to be perpendicular?
Find an equation of a line that is perpendicular to the line \(x=4\) that contains the point \((4,−5)\). Write the equation in slope-intercept form.
Answer
Find an equation of a line that is perpendicular to the line \(x=2\) that contains the point \((2,−1)\). Write the equation in slope-intercept form.
Answer
In Example, we used the point-slope form to find the equation. We could have looked at this in a different way.
We want to find a line that is perpendicular to \(x=5\) that contains the point \((3,−2)\). This graph shows us the line \(x=5\) and the point \((3,−2)\).
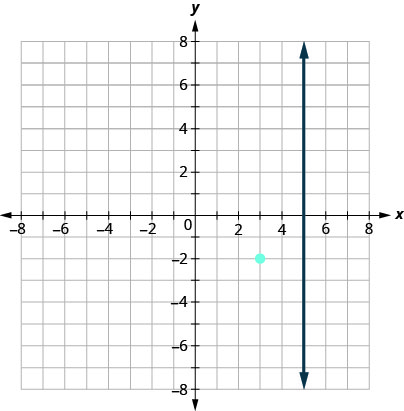 negative 8 to 8. The line goes through the points (5, 0), (5, 1), and (5, 2). The point (3, negative 2) is plotted. The line does not go through the point (3, negative 2)." />
negative 8 to 8. The line goes through the points (5, 0), (5, 1), and (5, 2). The point (3, negative 2) is plotted. The line does not go through the point (3, negative 2)." />
We know every line perpendicular to a vertical line is horizontal, so we will sketch the horizontal line through \((3,−2)\).
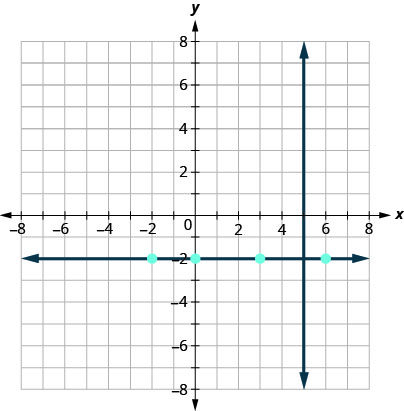 negative 8 to 8. The vertical line goes through the points (5, 0), (5, 1), and (5, 2). The horizontal line goes through the points (negative 2, negative 2), (0, negative 2), (3, negative 2), and (6, negative 2)." />
negative 8 to 8. The vertical line goes through the points (5, 0), (5, 1), and (5, 2). The horizontal line goes through the points (negative 2, negative 2), (0, negative 2), (3, negative 2), and (6, negative 2)." />
Do the lines appear perpendicular?
If we look at a few points on this horizontal line, we notice they all have y-coordinates of \(−2\). So, the equation of the line perpendicular to the vertical line \(x=5\) is \(y=−2\).
Find an equation of a line that is perpendicular to \(y=−3\) that contains the point \((−3,5)\). Write the equation in slope-intercept form.
Answer
The line \(y=−3\) is a horizontal line. Any line perpendicular to it must be vertical, in the form \(x=a\). Since the perpendicular line is vertical and passes through \((−3,5)\), every point on it has an x-coordinate of \(−3\). The equation of the perpendicular line is \(x=−3\).
You may want to sketch the lines. Do they appear perpendicular?
Find an equation of a line that is perpendicular to the line \(y=1\) that contains the point \((−5,1)\). Write the equation in slope-intercept form.
Answer
Find an equation of a line that is perpendicular to the line \(y=−5\) that contains the point \((−4,−5)\). Write the equation in slope-intercept form.
Answer
This page titled 3.5: Parallel and Perpendicular Lines is shared under a CC BY-NC-SA 4.0 license and was authored, remixed, and/or curated by Stanislav A. Trunov and Elizabeth J. Hale.